Note: This text has been automatically extracted via Optical Character Recognition (OCR) software.
Descriptive Geometry And Our Ancient Secrets.
Descriptive geometry makes use of two plans , one horizontal , the other vertical ; the object to be represented is then projected by means of perpendiculars dropped on these two planes , and one has thus two drawings , which are called the projections horizontal and vertical of the figure ; or in the case of a machine or building , the plan and elevation ; sections through fixed points are then addedand by means of the rule and compass the dimensions of each
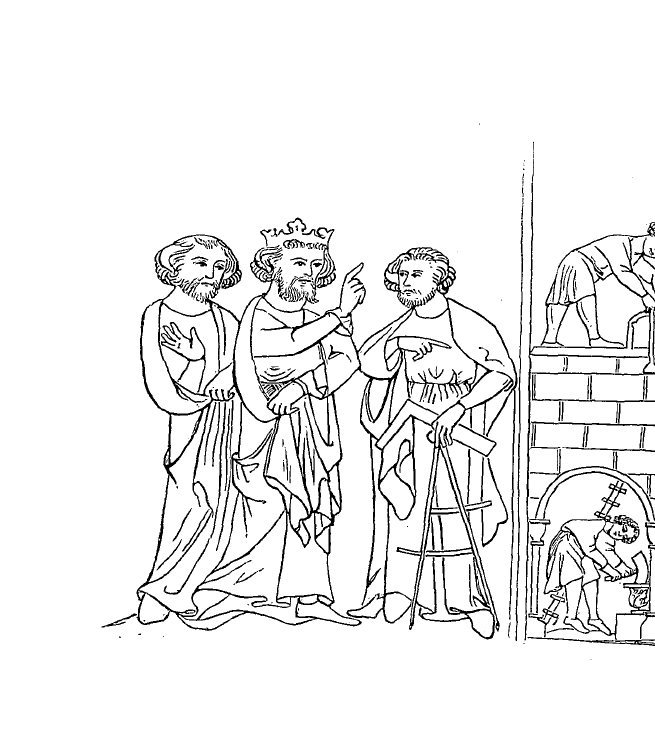
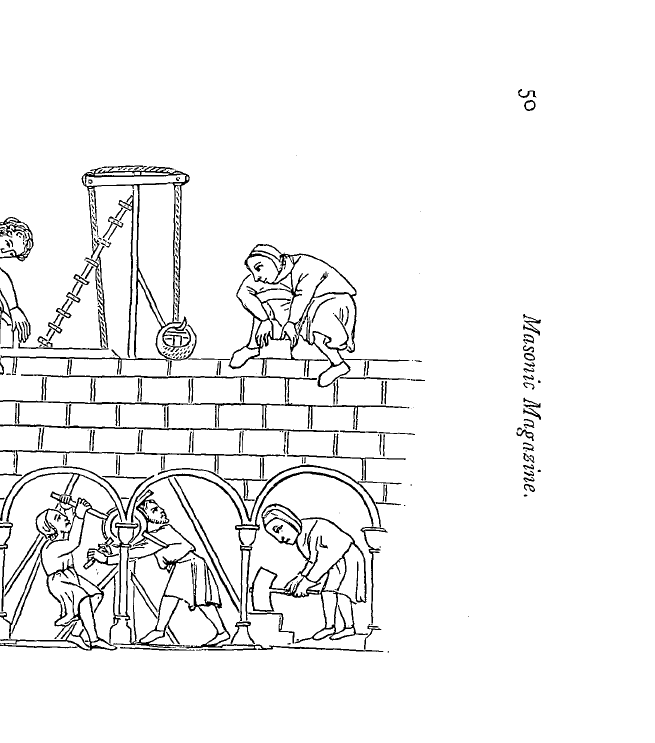
, stone , timber , or casting are determined and embodied in a pattern of wood or sheet metal , which serves to guide the workman . Such is the method that has always been used to determine the forms of stone which compose a building . The Greeks made use of these two planes under the names of " ichnographic " and " orthographic . " We read in the Bible of the construction of our Masonic temple : "And the house , when it was building , was built of stone made
ready before it was brought thither ; so that there was neither hammer , nor axe , nor any tool of iron heard in the house while it was building . '' Thus , then , the stones came ready cut out of the yard , the dimensions of each one having been previously worked out by the draughtsman . Moreover , tradition says that Hiram , the architect , was murdered by his workmen , because having discovered the use of the rule and compass he refused to publish his discovery . The secret was handed down from age to age as an occult science , and we see its symbols in the apron , trowel , square and compass of Freemasonry . The first treatise on the subject appeared about the middle of the sixteenth century , at a time when
architectural science was in its decay . This decay , indeed , probably accounts for the publication of what had previously been kept secret . The author of the work , Philibert de l'Orme , architect of the Tuileries , does not profess to be the discoverer of the system , but only to have extended its application . Other treatises on the subject appeared in the seventeenth and ei ghteenth centuries , where we first find the rules laid down for the construction of circular staircases ;
but for the most part the subject was not treated geometrically . The noble timber roofs of our cathedrals are examples of the use of the rule and compass in carpentry , on which a treatise was first published about the middle of the eighteenth century , and entitled " The Carpenter ' s Euclid . " However , the author allows that his treatment of the subject is only practical , and lacks rigour . The subject was raised to a science bthe great Mongeone of the
y , most brilliant of that body of learned men who presided at the birth of modern science under the enlightened encouragement of the first Napoleon . It was Monge who embodied , or shall I say re-embodied , the principles and rules which are common to the rigorous carrying- out of the different branches of the constructor ' s art ; he it was who united together what had been disconnected practical knowledge upon a harmonious and scientific basisunder the name of
, descriptive geometry . However , this science , put into shape b y Monge , did not assume under him the development which it has since attained . The growth of the railway system , for instance , has extended its applications , and it is descriptive geometry which has given the true solution of the form of the stones which compose a skew bridge .
It has often been a matter of speculation among Masonic students as to what were the real secrets of the mediaeval Masons . It is absurd to suppose that their guilds existed simply for the sake of a few words or grips , as in the case of the modern speculative society . Of course , we know that there was a certain amount of religious dogma taught in their lod ges ; this much we gather from the old constitutions left to us . But I am inclined to submit that the
science rediscovered by Monge , and called by him Descri ptive Geometry , constituted the real secret of our ancient brethren , and that it was this knowledge which they so carefully concealed from the profane , and which gave to them the monopol y which has immortalized their memory . Without enlarging upoir this point , I merely throw it out as a suggestion which , correct or not , certainly affords a reason and justification for the power wielded by the great mediaeval guilds of builders .
Note: This text has been automatically extracted via Optical Character Recognition (OCR) software.
Descriptive Geometry And Our Ancient Secrets.
Descriptive geometry makes use of two plans , one horizontal , the other vertical ; the object to be represented is then projected by means of perpendiculars dropped on these two planes , and one has thus two drawings , which are called the projections horizontal and vertical of the figure ; or in the case of a machine or building , the plan and elevation ; sections through fixed points are then addedand by means of the rule and compass the dimensions of each
, stone , timber , or casting are determined and embodied in a pattern of wood or sheet metal , which serves to guide the workman . Such is the method that has always been used to determine the forms of stone which compose a building . The Greeks made use of these two planes under the names of " ichnographic " and " orthographic . " We read in the Bible of the construction of our Masonic temple : "And the house , when it was building , was built of stone made
ready before it was brought thither ; so that there was neither hammer , nor axe , nor any tool of iron heard in the house while it was building . '' Thus , then , the stones came ready cut out of the yard , the dimensions of each one having been previously worked out by the draughtsman . Moreover , tradition says that Hiram , the architect , was murdered by his workmen , because having discovered the use of the rule and compass he refused to publish his discovery . The secret was handed down from age to age as an occult science , and we see its symbols in the apron , trowel , square and compass of Freemasonry . The first treatise on the subject appeared about the middle of the sixteenth century , at a time when
architectural science was in its decay . This decay , indeed , probably accounts for the publication of what had previously been kept secret . The author of the work , Philibert de l'Orme , architect of the Tuileries , does not profess to be the discoverer of the system , but only to have extended its application . Other treatises on the subject appeared in the seventeenth and ei ghteenth centuries , where we first find the rules laid down for the construction of circular staircases ;
but for the most part the subject was not treated geometrically . The noble timber roofs of our cathedrals are examples of the use of the rule and compass in carpentry , on which a treatise was first published about the middle of the eighteenth century , and entitled " The Carpenter ' s Euclid . " However , the author allows that his treatment of the subject is only practical , and lacks rigour . The subject was raised to a science bthe great Mongeone of the
y , most brilliant of that body of learned men who presided at the birth of modern science under the enlightened encouragement of the first Napoleon . It was Monge who embodied , or shall I say re-embodied , the principles and rules which are common to the rigorous carrying- out of the different branches of the constructor ' s art ; he it was who united together what had been disconnected practical knowledge upon a harmonious and scientific basisunder the name of
, descriptive geometry . However , this science , put into shape b y Monge , did not assume under him the development which it has since attained . The growth of the railway system , for instance , has extended its applications , and it is descriptive geometry which has given the true solution of the form of the stones which compose a skew bridge .
It has often been a matter of speculation among Masonic students as to what were the real secrets of the mediaeval Masons . It is absurd to suppose that their guilds existed simply for the sake of a few words or grips , as in the case of the modern speculative society . Of course , we know that there was a certain amount of religious dogma taught in their lod ges ; this much we gather from the old constitutions left to us . But I am inclined to submit that the
science rediscovered by Monge , and called by him Descri ptive Geometry , constituted the real secret of our ancient brethren , and that it was this knowledge which they so carefully concealed from the profane , and which gave to them the monopol y which has immortalized their memory . Without enlarging upoir this point , I merely throw it out as a suggestion which , correct or not , certainly affords a reason and justification for the power wielded by the great mediaeval guilds of builders .